ROTATIONAL DYNAMICS: EXERCISES
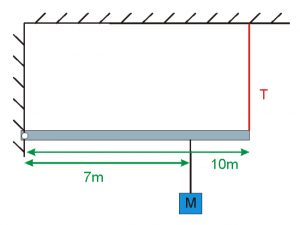 A mass M = 120 kg hangs from a beam which is held by a cable.
A mass M = 120 kg hangs from a beam which is held by a cable.
- What is the tension T in the cable?
- Does the wall exert any force on the beam? Which one?
- A stone of mass 0.6 kg is forced to travel in a circle while it hangs from the ce
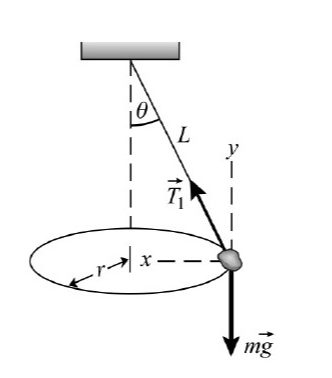 iling, as shown in Figure. If the angle between the rope and the vertical is θ = 30º and the radius of the circle is r = 35 cm:
iling, as shown in Figure. If the angle between the rope and the vertical is θ = 30º and the radius of the circle is r = 35 cm:
- What is the speed of the stone?
- What is the tension in the rope?
- A wheel of radius r = 80 cm has moment of inertia 10 kg m2. It is rotating around its central axis propelled by a rocket attached to a point on its outer rim. The rocket is expelling gas
tangentially to the wheel, resulting in a constant force. Determine:- The magnitude of the equivalent force, if we know that the wheel, starting from rest, reaches an angular speed of 1 rev/s in 6 s.
- The value of both tangential and normal acceleration in a point on the outer rim of the wheel.
- The angle that the total acceleration forms with the radius at that point.
- The time that the wheel takes to reach the same angular velocity, under the action of the same force, if we add a very thin ring of mass 5 kg around the outer rim.